Pedal Triangles

By

Cassian Mosha

Question: Prove the pedal triangle of the pedal triangle of the pedal triangle of a point is similar to the original triangle. That is, show that the pedal triangle A'B'C' of pedal triangle RST of the pedal triangle XYZ of pedal point P is similar to triangle ABC.

Let’s construct a triangle ABC. Then using script tool let's construct a pedal triangle XYZ. Then using the same point let's construct anaother pedal triangle RST.

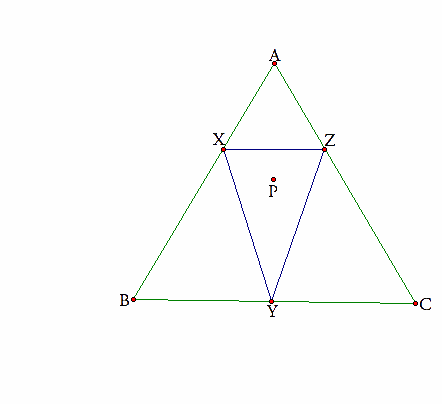
Figure 1

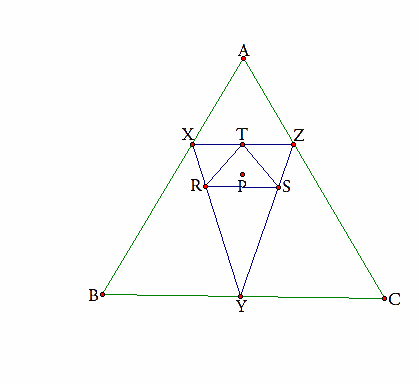
Figure 2

Let's again construct another pedal triangle A'B'C'using the same point P, and claim that triangle ABC is similar to triangle A'B'C'. We will need to show that these two triangles are similar

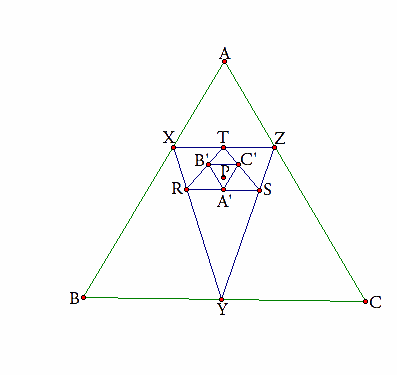
We can show the similarities of the two triangles by comparing the ratio of the sides. As stated in Euclidean geomerty that the ratio of the lenghts of corresponding sides of a similar triangle is constant that is if triangle ABC is similar to triangle A'B'C' then AB/A'B'=BC/B'C'=AC/A'C'

Proof:

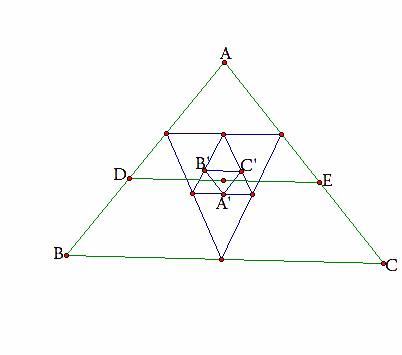
If AB is similar to A'B', then triangle ABC is similar to triangle A'B'C' by ASA and each quotient as shown above is 1. Let us assume that AB is not equal to A'B'. Suppose that AB<A'B' and the case AB>A'B' is similar and let D be a point on line AB such that A'B'~AD. Also let L be a line through D parallel to BC. By Pasch's theorem L intersects AC at a point E. Angle ADE is similar to angle ABC and similar to angle A'B'C' Thus triangle ABC is similar to triangle A'B'C' by ASA, thus AD/ab=AE/AC. Similarly A'B'/AB=B'C'/BC. Done.

Return